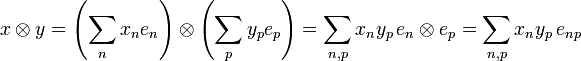GoI for MELL: exponentials
From LLWiki
Revision as of 12:20, 5 June 2010 by Laurent Regnier (Talk | contribs)
The tensor product of Hilbert spaces
Recall that 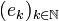 is the canonical basis of
is the canonical basis of 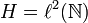 . The space
. The space 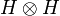 is the collection of sequences
is the collection of sequences 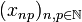 of complex numbers such that:
of complex numbers such that:
| ∑ | | xnp | 2 |
| n,p |
converges. The scalar product is defined just as before:
-
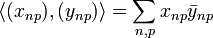 .
.
If 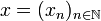 and
and 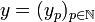 are vectors in H then their tensor is the sequence:
are vectors in H then their tensor is the sequence:
-
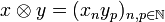 .
.
In particular if we define: 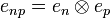 so that enp is the (doubly indexed) sequence of complex numbers given by enpij = δniδpj then (enp) is a hilbertian basis of
so that enp is the (doubly indexed) sequence of complex numbers given by enpij = δniδpj then (enp) is a hilbertian basis of 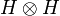 : the sequence x = (xnp) may be written:
: the sequence x = (xnp) may be written:
-
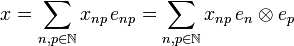 .
.
By bilinearity of tensor we have: